


Guilhem MOLLON
guilhem.mollon@insa-lyon.fr
Associate Professor
National Institute for Applied Sciences of Lyon
PhD, Civil Engineering, INSA Lyon
HDR, INSA Lyon



Guilhem MOLLON
guilhem.mollon@insa-lyon.fr
Associate Professor
National Institute for Applied Sciences of Lyon
PhD, Civil Engineering, INSA Lyon
HDR, INSA Lyon
In the framework of the development of mountain areas, predictive tools are necessary to assess the risk related to rock avalanches. The current advances in computation power make it possible to imagine a numerical simulation method of the propagation of a rock mass along a natural slope, using the Discrete Elements Method (DEM). This method is based on an explicit resolution scheme of the equations of motion, combined with contact laws which prescribe the repulsive forces that appear between dicrete blocks in case of interpenetration. In the chosen strategy, each block of an avalanche is modeled by a spheropolyhedron, which highly simplifies the neighbours and contacts detection as well as the computation of the repulsive forces. In the case of rock avalanches (and more generally of granular flows), it appeared interesting to pay a lot of attention to the bloc-shapes, and to simplify as much as possible the contact law. Indeed, it seems difficult to reproduce in all their complexity all the phenomena which occur during any of the numerous impacts within the flow (which justifies the adoption of a simple contact law in order to consider it as an "energetic black box" with relevant results at the scale of the whole flow), whereas the sizes and shapes of the blocks are important parameters of the flow. A four-parameters law (normal and tangential contact stiffnesses, friction coefficient, and normal restitution coefficient) was thus chosen in order to have a good control over the different sources of energy dissipations within the flow.
Before considering a real case in a natural context, the relevance of the model was evaluated by confronting it to the experimental results published by Manzella and Labiouse (2009). Their experiments consisted in realizing a packing of small bricks (6000 to 10000, each one of them being 3cm long), and to release this assembly on an inclined plane in order to observe the granular flow on the slope and its deposition on the horizontal plane. The estimation of the contact parameters was performed using the results of additionnal experiments using the same materials as in the original experiments by manzella and Labiouse (bricks and plastic support). Several collision tests were performed and shot by two high-speed cameras. A back-analysis of the trajectories of falls and rebounds allowed to define a set of 8 contact parameters (4 parameters for the brick-support contact, and 4 for the brick-brick contact), which in turn were introduced in a simulation of the whole avalanche event. The results of this simulation (velocity of propagation and deposition, shape and size of the granular deposit) showed a very satisfactory correspondence with the experimental results, and proved that the collective behavior of a granular mass can be reproduced from very simple contact laws calibrated on isolated impacts.
On-going work is related to a future application in a natural context (i.e. to a real event of rock avalanche), and on the new levels of complexity that such a simulation implies (topography, soft subtrate, rock fracturation, etc.)
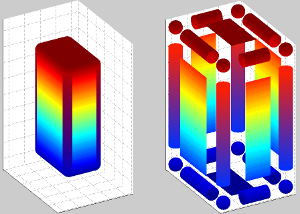
Figure 1. Strategy of shape representation by spheropolyhedron: each vertice is replaced by a sphere, and each edge by a cylinder.
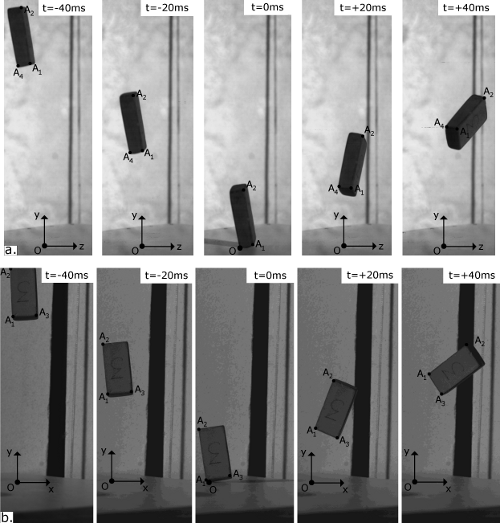
Figure 3. Same collision experiment shot by two high-speed cameras (a. et b.).
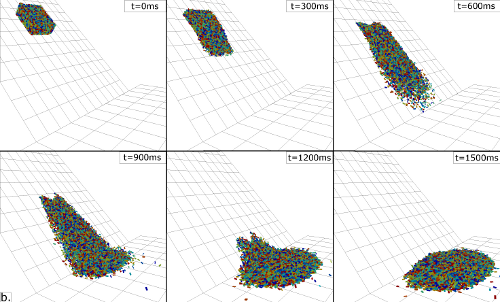
Figure 5. Simulation of the experiment published by Manzella and Labiouse (2009), from the packing in the starting box to the final deposit on the horizontal plane.
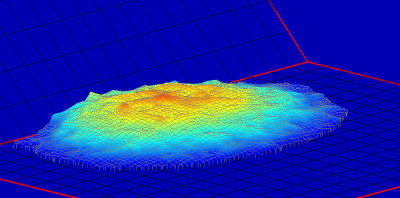
Figure 7. Non-convex envelope of the final deposit, giving access for example to its volume and solid fraction.

Figure 2. Simple contact law with four parameters. The energy dissipation is introduced by classical Coulomb friction, and by a coefficient of normal dissipation describing the ratio between the loading and unloading stiffnesses.
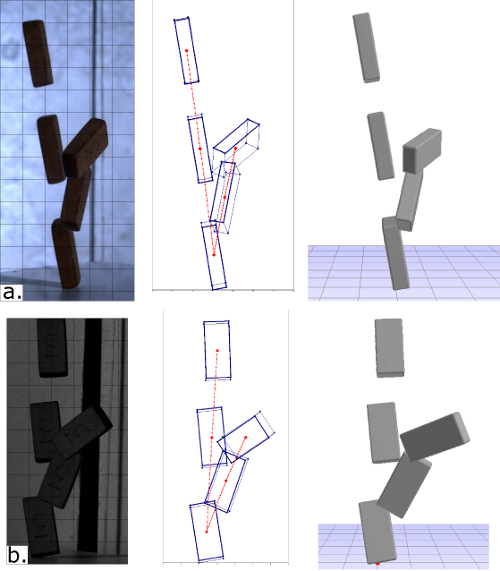
Figure 4. Determination of the contact parameters by back-analysis on the two cameras (a. et b.). From left to right : shooting, 3D determination of the trajectory, best numerical reproduction of the impact.
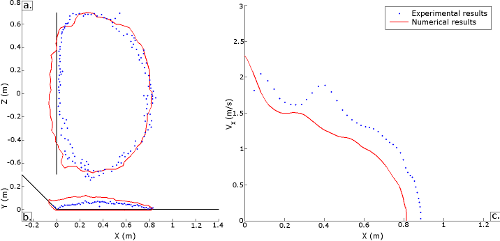
Figure 6. Comparison between the experimental and numerical results, in terms of deposit shapes (a. et b.) and of avalanche front velocity (c.). The results are considered very satisfactory provided that the contact parameters were calibrated on additional tests, and not by back-analysis of the whole event.
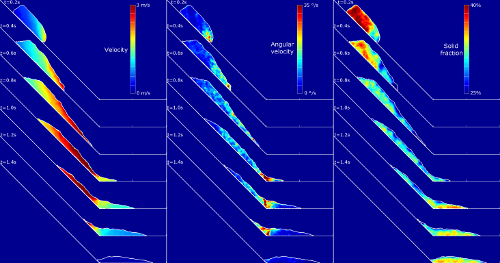
Figure 8. Interpolation techniques utilized to plot continuous fields of some interesting quantities, such as velocities and angular velocities of the particles or local solid fraction of the granular mass.